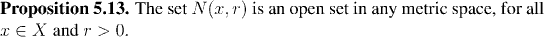arrow_back arrow_forward

Class Contents

• Open and closed sets

Open and Closed Sets

We shall extend our defintion of open and closed sets to general metric spaces. Once we've done this, the ideas of openness and closedness will provide perfect tools for studying important properties of sets. Two such are connectedness (whether a set falls naturally into several pieces or not) and compactness. We shall study connectedness towards the end of the present chapter, and compactness and its applications will occupy all of the next chapter.

Recall the defintion of open set from Chapter 2. In a metric space, it makes sense to use a slightly different defintion, which is tantamount to the same thing in the real numbers. You should recognize the defintion we use here as an extension of the equivalent definition of open sets from Homework 2.

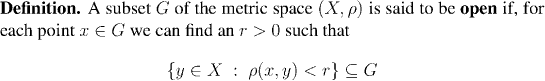
A convenient notation is to call a set of the form

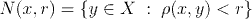
an open ball centered on x, of radius r. Thus a set is open if given any point we can always find an open ball of some positive radius centered on that point, which is contained in the set.

Work an interactive exploration of the definition of open sets

The definition of a closed set in a metric space is a staightforward extension of the defintion from Chapter 3

Many of the results which we prove for open and closed sets in this section will be familar as analogues of results which we have already seen for open or closed subsets of the real numbers. The next proposition is a direct extension of Theorem 3.14.

Proof.

The very first example involving open sets that we saw was that the open intervals in the real line are open sets. The next result is in the same spirit. However, because of the slightly different definition of open set in general, the proof is not absolutely immediate.

Proof.

One of the important uses for open sets in metric spaces is the fact that we can determine whether or not a function is continuous entirely in terms of how it maps open sets. This characterization of continuity is completely different from the epsilon-delta definition. In fact it is remarkable that we can determine whether or not a function is continuous without involving any limits in the defintion at all.

Before we can come to this result, howver, we need to define two sets related to a given function.