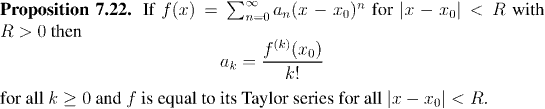arrow_back arrow_forward

Class Contents

• Taylor series
  • General power series
  • Higher order derivatives
  • Differentiating power series
  • Power series are unique

Taylor Series

General Power Series

All of the power series that we have studied so far have been of the form

and we showed that they converge inside disks centered on zero, and diverge outside those disks. However, we can easily do a change of variable, to study power series of the form

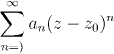
where z₀ is fixed. This series converges on a set of the form

which is a disk of radius R centered on z₀.

In this section we shall be interested in real-valued functions of a real variable which come from power series. The power series will be of the form

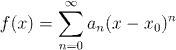
where x and x₀ are real numbers. The region in the real line where this series converges is the interval (x_0-R, x_0+R), which is called the interval of convergence (it is just the intersection of the disk of radius R centered on x₀ with the real line).

Higher Order Derivatives

In Chapter 4 we discussed functions which have a derivative at every point an interval (a,b). If f(x) is such a function, it is said to be differentiable on (a,b) and its derivative is f'.

Now it may be that the function f' is also differentiable on (a,b) and, if so, then its derivative, f'' is called the second derivative of f. By the same token, if f'' is differentiable on (a,b) then its derivative is called the third derivative of f on (a,b). In general, if we can repeat this process k times then we say that f is k-times differentiable, and that the last function obtained in this way by repeated differentiation is the kth derivative of f.

Warning:

Just as there is no reason why an arbitrary function should be differentiable at all, so there is no reason, just because a function can be differentiated k times, to assume that it can be differentiated k+1 times!

Notation:

We write

for the kth derivative of f, if it exists. By convention we say

Differentiating Power Series

References: Theorem 7.9 | Lemma 7.12

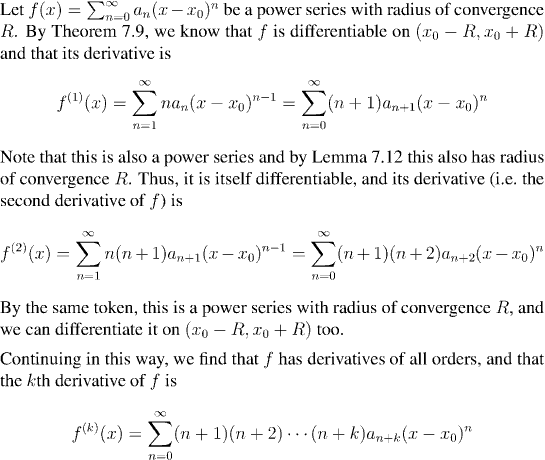
If

is a power series, then recall that f(x₀)=a₀. In other words, the value of the power series at x₀ is equal to the first coefficient of the series. Taking this principle to the power series we found for the derivatives of f, we find that

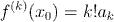
Conversely,

Thus,

We have shown that the values of the derivatives of f at just one point (namely x₀) determine the values of f throughout the whole interval of convergence. When f can be expressed by a series of this kind, we call it a Taylor series.

We can summarize the results of the last couple of sections as:

It may not seem like a great revelation that f can be expressed as a power series in terms of its derivatives. After all, if we know all the derivatives of a function we already have to know a whole lot about it. There are however some very nice payoffs from the observations of the last few paragraphs.

Power Series are Unique

A function cannot be expressed as a power series in more than one way. Put more precisely, if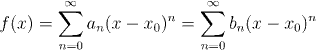

for all x in a non-empty open interval, then a_n=b_n for all n.

Question: Can you see why this is?